Table of Contents
Diameter Range
Circular waveguides’ diameter is a very important parameter that has a direct impact on the efficiency of the use of waveguides for various applications in microwave and radio frequency communication. Circular waveguides can be used in one or another diameter. The minimal and maximal diameter can be usually equal to 10 mm and 100 mm. However, the selection of this parameter is strictly connected to the operational frequency band. For example, the diameter of 10 mm can be sufficient for the operational frequencies in the V band, approximately 40 GHz to 75 GHz. At the same time, the lowering of Attenuation is what is required for the efficient use of waveguides.
This can be well-achieved with the use of larger diameters of 100 mm. However, these waveguides’ diameter can be useful for the lower operational frequency, i.e. the L band, 1 GHz to 2 GHz. The increased diameter can be useful for reduction of the cutoff frequency, which is crucial for these lower operations frequencies. These life sizes ensure that the waveguide can support the propagation of the signals in the mode wave required for the transmission of the signal with minimum losses.
The transmission efficiency is also closely connected to the material selected for the waveguide. For example, brass is the most popular material for waveguides with applied power up to several kilowatts. The reason is that Brass has a good conductivity and mechanical strength. At the same time, for airborne systems, the preferred material is Aluminum since its weight is significantly less.
EIA Standard Sizes
The Electric Industries Alliance standardizes dimensions for circular waveguides to facilitate design and ensure they will be compatible with different systems. Several EIA standard sizes are available to help engineers quickly select the best waveguide performance required for their applications. Thus, there are many standard sizes depending on the application. However, EIA specifies several common sizes, where common sizes defined by the EIA are 19.05 mm , 31.75 mm , and 50.8 mm.
Standard sizes ensure that a component will be compatible with installations on satellites and operation alongside various ground-based radar operations. The use of these sizes is particularly helpful in reducing production costs because one size can be used throughout the design process. For instance, if a company produced waveguides for satellite communication, the organization would consider the 50.8 mm waveguide to offer the cutoff frequency necessary to avoid signal loss. With a higher cutoff frequency than other options, larger dimensions ensure that signal loss is minimal. In contrast, a smaller 19.05 mm diameter waveguide might be used for medical image analysis device because the device needs to use a higher frequency to deliver more detailed imaging results.
EIA sizes facilitate the design process because they are already validated. By their nature, these sizes have already been pre-validated and will pass any industry requirements. Thus, manufacturers do not need to worry about taking the time to develop these standards and test them to ensure functionality. As for the production sector, it ensures the right standards are being used for a constant production cycle, meaning that parts will be plated and punched in batches ready for construction in their product application. The future of these parts will then be more reliably delivered without validation issues in shorter timeframes.
Cutoff Frequency Relation
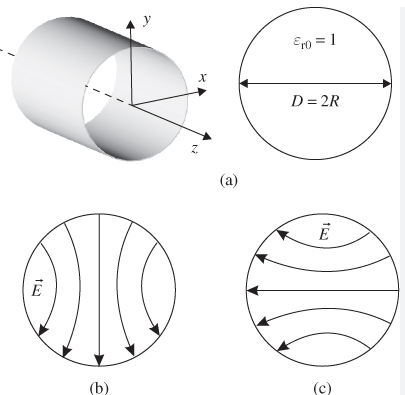
The waveguide’s cutoff frequency is a particularly important feature because it determines the facility with which the device can transmit microwave and radio frequencies. The relationship between the diameter of the waveguide and its cutoff frequency is defined as follows:
fc=1.841c2πaf_c = \frac{1.841c}{2\pi a}fc=2πa1.841c
In the formula above, fcf_c is the cutoff frequency of the waveguide. The characteristics of the waveguide are defined by the value 1.841, which, when interwoven in the formula, describes the first root of the Bessel’s function of the first kind of order one for the TE11 mode. ccc can be approximated as 3×1083 \times 10^83×108 meters per second. Assuming that the waveguide has a diameter of 2 cm, the cutoff frequency for the TE11 mode would equal to 2.78 GHz. At the same time, a waveguide diameter of 10 cm would have the cutoff frequency of 556 MHz, which attests to the inverse relationship between these values.
The necessity of the established relationship can be highlighted by way of reference to aerospace designs. In these situations, waves of a higher frequency are typically used in order to obtain more detailed information. Suppose that the engineers opt to use an image wave with 5 GHz resonating through the TE11 mode and desire that the waves be transmitted evenly to the other end of the chamber. The changes registered at the other end will mirror the condition of the investigated object.
The designers will have to proportionally reduce the diameter of the waveguide in order to maintain a high cutoff frequency. A similar conclusion can be made in regard to telecommunication systems. By minimizing the size of the waveguide, the engineer can ensure that the cutoff frequency can correspond to the exact frequency at which data transmission occurs. This achievement is particularly important over long distances, and the established rules apply to the construction of transcontinental and transoceanic data transfer systems in the same manner as to the aforementioned aerospace devices.
Frequency Band Usage
The choice of circular waveguide dimensions has the strongest influence on their operational frequency bands and, therefore, their suitability for certain applications. Moreover, the size of the waveguide can also affect its loss coefficient and efficacy with various frequencies. For instance, in satellite communication and radar systems, engineers will typically use smaller waveguides for high-frequency applications, such as the Ka-band.
At this band, the nominal frequency ranges from 26.5 to 40 GHz. These applications require smaller diameters to protect a high cutoff frequency and, ideally, decrease losses. At such a frequency, which is equal to 30 GHz in practical applications, a waveguide with a diameter of approximately 31 mm or 3.1 cm will be the best solution. It is adequately small to provide the necessary high cutoff frequency and large enough diameter to decrease losses.
Conversely, for applications that use medium-sized waveguides in the range of 1-2 GHz, such as a signal from a GPS or some telecommunications systems, the L-band, and a waveguide with a 2-10 cm diameter may be a suitable solution. In this case, the waveguide’s diameter will need to be significant to reduce its cutoff frequency while ensuring medium loss coefficients. A diameter of 100 mm or 10 cm will be the optimal solution for this frequency range.
By using an incorrectly sized waveguide, the company essentially increases the operational cost of its product by causing more power to the given device to develop the same level of waveguide losses. In conclusion, in my project, I would have used a medium-sized waveguide with a 100 mm diameter for making the radar at a given X-band. At this frequency, it most likely would satisfy the requirements for a low loss coefficient while keeping the cutoff frequency at a moderate level to ensure unparalleled efficacy at an airport.
Precision and Tolerance
The precision and tolerance in the dimensions of a circular waveguide are crucial to success in such applications as satellite communications and precision radar systems. Given the stringent requirements of the applications in question, the waveguide’s manufacturer has to comply strictly with the identified tolerances, as even the smallest divergence can disrupt the process. The notion of the precision of a waveguide width implies the proximity of the dimensions of the samples produced by the actual sample, whereas the tolerance is the deviation of these two measurements.
The tolerance for the X-band waveguide fillet made of brass and aluminum would be equal to 0.02 mm. The C-band waveguide for telecommunications tools made of the same materials requires the tolerance of 0.05 mm, as the physical and chemical properties of the materials in combination with the reduced appurtenant frequency make the waveguide less prone to disruptions if the dimensions of the channel are either smaller or larger than normal.
Higher precision and a stricter tolerance are required as the frequency involved increases. Less tolerance should be allowed because even a slight decrease in the diameter of the waveguide would lead to a significant increase in the cutoff frequency, which, in turn, will prevent the system from operating at the expected efficiency rate. Low tolerances, such as 0.02 mm for the X-band waveguide, are required when dealing with extremely high frequencies due to the relatively low radius, which will cause the cutoff frequency to be significantly affected by the level of deviation.
In turn, the tolerance of 0.05 mm for the C-band is allowed due to the frequency being lower, which will allow the cutoff frequency to be influenced to a lesser degree by the waveguide channel’s dimensions. The designated limit of 0.02 mm is an example of the highest floor, whereas the other provided dimension is the lowest ceiling limit. Notably, the considered high-tolerance level for the waveguide in the C-band would still positively impact the system’s operational efficiency and its longevity.
It is important to note that the use of the CNC machine would be required in most cases to maintain the high level of precision described above. The tight tolerance and, therefore, strict requirements will be connected to the expenses, not just in terms of the material but also due to the increased level of skill required as waveguide’s production will be handled on a particular machine. Practice shows, though, that the benefits of maintaining the designated level of precision above are worth the price, as considerable expenses connected to the tools and equipment for telecommunications and precision radar systems and satellites are involved.
Material and Wall Thickness
There are several crucial factors that affect the performance of circular waveguides, the choice of material and wall thickness among them. Different material and thickness cater to distinct needs, based on location, transmitter frequency, power, and other specifications. Regarding their operation, the most critical characteristics of a waveguide are its ability to conduct power, transmit the desired frequency, and maintain a functional state for extended periods of time. When discussing a material, it is important to note that the choice of metal is most commonly limited to copper, brass, or aluminum.
Each of these metals has a unique configuration of characteristics that make them most useful in distinct situations. Copper is ideal for high-frequency use because of its excellent electrical conductivity, which enables minimal signal loss. In applications where signal integrity is critical, such as broadcasting, copper waveguides are instrumental. Aluminum’s weight advantage over copper also makes it the metal of choice in aerospace, where weight must be minimized.
The material’s slightly poorer conductivity is a minor disadvantage at lower power levels, where its lightness far outweighs the modest decrease in conductivity. Brass is less commonly used due to its lack of advantageous characteristics — it is heavier than aluminum yet less conductive than copper. There are few applications where brass is the optimal desision based on the material alone.
The thickness of the wall is also a significant factor in a circular waveguides ability to conduct power, handle physical stress, and humidity. For example, a relatively high value of 2-3 mm might be the best choice for satellite uplinks, where the waveguide must withstand several kilowatts of power. The maintenance of sufficient thickness allows it to ensure that electric field inside it does not lead to leaks nor that it is destroyed by the generated heat. On the other hand, for another type of waveguide, deployed indoors and operating at only a few tens of watts, using a 1 mm wall would be sufficient. Such a thickness would save material and keep the waveguide cheap and light.
Choosing an appropriate material and wall thickness is a matter of cost-efficiency and complying with the technical requirements. As a generic example, aluminum’s worst case scenario is entirely acceptable in the majority of applications that do not require extreme conductivity — switching to copper would be wastefully expensive and not result in better performance.